-
 Home
Home
-
 News
News
-
 Colleges
Colleges
Pakistan's Largest Database of Colleges and Universities
Explore Largest Directory of Private and Govt. Colleges, Universities and find best institute for your future Education.
-
 Courses
Courses
-
 Admission
Admission
-
 Lectures
Lectures
-
 Online Test
Online Test
Short Question
- 9th Class Physics Short Questions
- 9th Class Chemistry Short Questions
- 9th Class Math Short Questions
- 9th Class Biology Short Questions
- 9th Class Computer Short Questions
- 9th Class English Short Questions
- 10th Class Physics Short Question
- 10th Class Chemistry Short Question
- 10th Class Math Short Question
- 10th Class Biology Short Question
- 10th Class Computer Short Question
- 10th Class English Short Question
-
 Past Papers
Past Papers
-
 Date Sheets
Date Sheets
-
 Results
Results
Exam Results 2024
Check online Results 2024 Matric Inter BA BSc B.Com MA MSc M.Com CSS PCS MCAT ECAT of all educational boards and universities in Pakistan
-
 Study Abroad
Study Abroad
Study Abroad Programs and Opportunities for Pakistani Students
Explore free study abroad search to find programs, consultants, events to study in USA, UK, Australia, China, Malaysia and many others.
-
 Jobs
Jobs
-
 Tutors
Tutors
-
 More
More
-
 Apps
Apps
This online test contains MCQs about following topics:
Introduction - Solutions of Equations Reducible to the Quadratic Equation - Three Cube Roots of Unity - Four Fourth Roots of Unity - Polynomial Function - Theorems - Synthetic Division - Relations between the Roots and the Coefficients of a Quadratic Equation - Formation of an Equation whose Roots are given - Nature of the Roots of Quadratic Equation - System of two Equations Involving two Variables - Problems on Quadratic Equationsa
FA Part 1 Mathematics Ch. 4 Test

FA Part 1 English Medium Subjects
FA Part 1 Urdu Medium Subjects
Online MCQ`s Test For Chapter 4 "11th Class FA Mathematics Chapter 4 Online Test"
Try The MCQ`s Test For Chapter 4 "11th Class FA Mathematics Chapter 4 Online Test"
-
Total Questions20
-
Time Allowed30
Question # 1
If the sum of the roots of the equation kx2 - 2x + 2k = 0 is equal to their product, then the value of k is:
Question # 2
If P(x) is a polynomial of degree m and Q(x) is a polynomial of degree n, the quotient P(x) + Q(x) will produce a polynomial of degree:
Question # 3
If a polynomial P(x) = x2 + 4x2 - 2x + 5 is divided by x - 1, then the reminder is:
Question # 4
Solution set of the equation x2 - 3x + 2 = 0 is
Question # 5
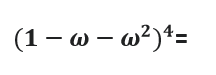
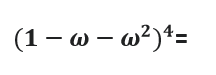
Question # 6
For what value of k, the sum of the roots of the equation x2 + kx + 4 = 0 is equal to the product of its roots:
Question # 7
One of the roots of the equation 3x2 + 2x + k = 0 is the reciprocal of the other, then k = ...............:
Question # 8
If one root of 2x2 + ax + 6 = 0 is 2 then the value of a is:
Question # 9
For what value of k, the roots of the equation x2 + √k x + 2 = 0 are equal:
Question # 10
If the roots of x2 - bx + c = 0 are two consecutive integers, then: b2 - 4ac =
Question # 11
If the Discriminant of a quadratic equation is a perfect square, then roots are:
Question # 12
Four fourth roots of 625 are:
Question # 13
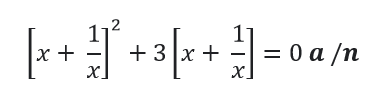
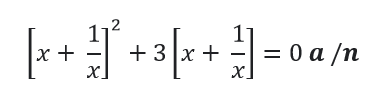
Question # 14
Sum of all three cube roots of unity is:
Question # 15
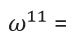
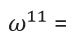
Question # 16
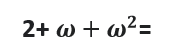
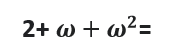
Question # 17
Which one is radical equation:
Question # 18
How many complex cube roots of unity are there:
Question # 19
If P(x) is a polynomial of degree m and Q(x) is a polynomial of degree n, the product P(x) . Q(x) will be a polynomial of degree:
Question # 20
FA Part 1 Mathematics Chapter 4 Test
Here you can prepare FA Part 1 Mathematics Chapter 4 Quadratic Equations Test. Click the button for 100% free full practice test.
Top Scorers Of Chapter 4 "11th Class FA Mathematics Chapter 4 Online Test" MCQ`s Test
-
H Hazel Lucy 30 - Jun - 2024 01 Min 27 Sec 20/20 -
A Ayesha Zahid 19 - Sep - 2024 02 Min 59 Sec 20/20 -
M Mahad Awan 14 - Apr - 2024 00 Min 21 Sec 18/20 -
T Tayyaba Sohail 11 - Feb - 2024 01 Min 31 Sec 16/20 -
G Ghufran 11 - Jan - 2024 06 Min 27 Sec 16/20 -
S Sana Arshad 11 - Oct - 2024 04 Min 27 Sec 15/20 -
H Hina Amir 19 - Aug - 2024 06 Min 40 Sec 15/20 -
T Taina Obery 21 - Feb - 2024 05 Min 06 Sec 14/20 -
C Celebrities Zulnnurain17 20 - Mar - 2024 10 Min 45 Sec 14/20 -
Z Zarnab Ali 29 - Sep - 2024 04 Min 10 Sec 13/20 -
H hamid butt 13 - Feb - 2024 03 Min 06 Sec 12/20 -
N Noor ul ain Zahid 04 - Sep - 2024 11 Min 03 Sec 12/20 -
A Alex Olliver 14 - Feb - 2024 11 Min 17 Sec 12/20 -
B Butt Abeer 29 - Nov - 2024 13 Min 11 Sec 12/20 -
A Aqeel Akram 22 - Feb - 2024 03 Min 54 Sec 11/20

.gif)